Laplace Transform

Inverse Laplace Transform

Given a “nice” function $f(t)$ and its Laplace transform $F(s)$, we have $f(t)=\frac{1}{2\pi j}{\int }_{\alpha -j\infty }^{\alpha +j\infty }F(s)e^{st}ds$ where $\alpha$ is such the vertical line lies in the radius of convergence of the Laplace Transform $F(s)$, $F(s)$.

Note that:

• The above is a contour integral in the complex plane! The above is “sum of exponentials” Prof talked about!!

We are not taught how to evaluate these… so we will use partial-fraction decomposition and look up the inverse transforms in a table.

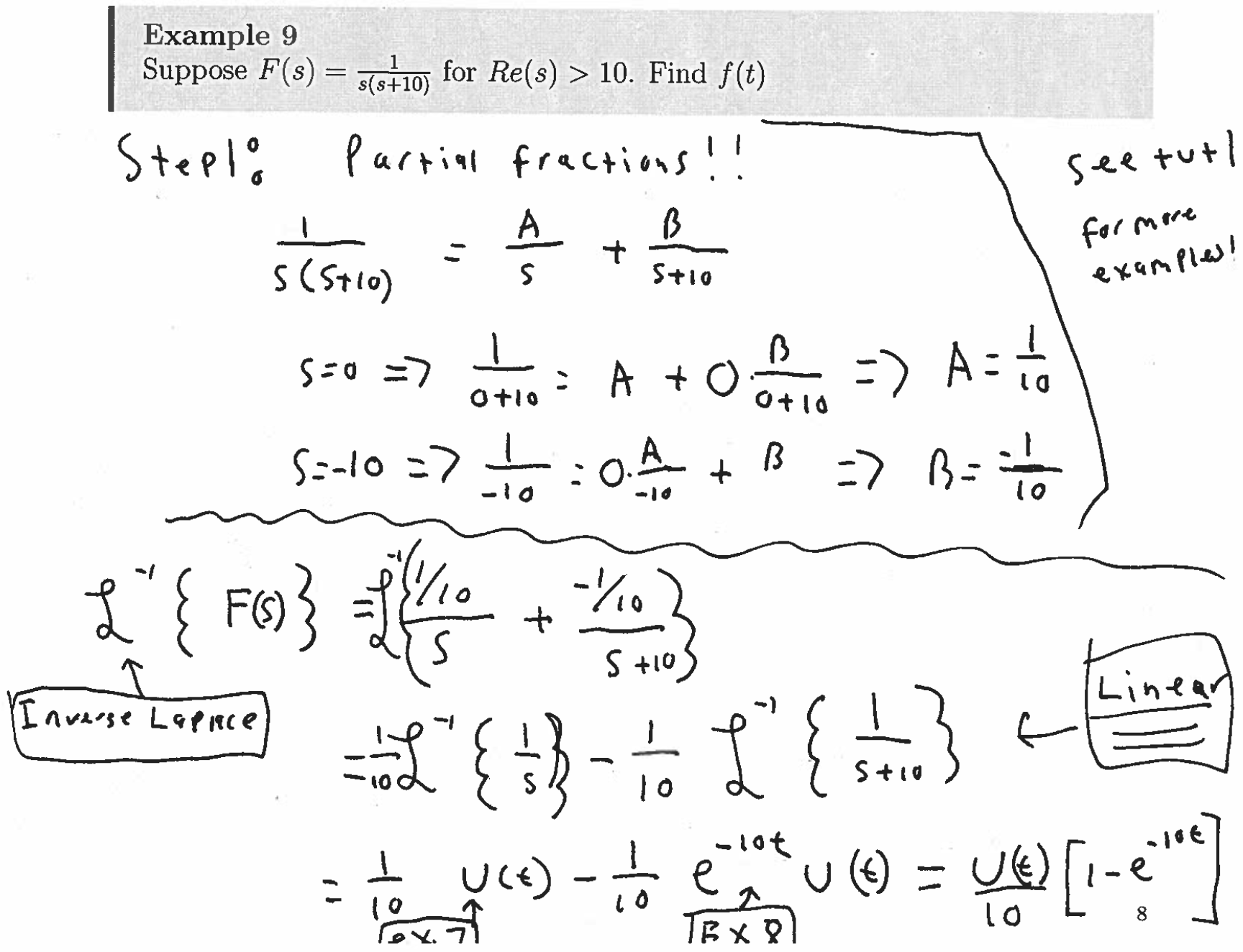
Lecture 7

We care about these ideas because the inverse Laplace transform is a complex valued integral and because of this, we can compute the inverse transform of $F(s)$ by only knowing information about the poles of $F(s)$.

Two important results that are related to poles:

Summary